First Hardy–Littlewood conjecture
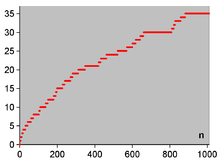 Plot showing the number of twin primes less than a given n. The first Hardy–Littlewood conjecture predicts there are infinitely many of these. | |
| Field | Number theory |
|---|---|
| Conjectured by | G. H. Hardy John Edensor Littlewood |
| Conjectured in | 1923 |
| Open problem | yes |
In number theory, the first Hardy–Littlewood conjecture[1] states the asymptotic formula for the number of prime k-tuples less than a given magnitude by generalizing the prime number theorem. It was first proposed by G. H. Hardy and John Edensor Littlewood in 1923.[2]
Statement
[edit]Let be positive even integers such that the numbers of the sequence do not form a complete residue class with respect to any prime and let denote the number of primes less than st. are all prime. Then[1][3]
where
is a product over odd primes and denotes the number of distinct residues of modulo .
The case and is related to the twin prime conjecture. Specifically if denotes the number of twin primes less than n then
where
is the twin prime constant.[3]
Skewes' number
[edit]The Skewes' numbers for prime k-tuples are an extension of the definition of Skewes' number to prime k-tuples based on the first Hardy–Littlewood conjecture. The first prime p that violates the Hardy–Littlewood inequality for the k-tuple P, i.e., such that
(if such a prime exists) is the Skewes number for P.[3]
Consequences
[edit]The conjecture has been shown to be inconsistent with the second Hardy–Littlewood conjecture.[4]
Generalizations
[edit]The Bateman–Horn conjecture generalizes the first Hardy–Littlewood conjecture to polynomials of degree higher than 1.[1]
Notes
[edit]- ^ a b c Aletheia-Zomlefer, Fukshansky & Garcia 2020.
- ^ Hardy, G. H.; Littlewood, J. E. (1923). "Some Problems of 'Partitio Numerorum.' III. On the Expression of a Number as a Sum of Primes". Acta Math. 44 (44): 1–70. doi:10.1007/BF02403921..
- ^ a b c Tóth 2019.
- ^ Richards, Ian (1974). "On the Incompatibility of Two Conjectures Concerning Primes". Bull. Amer. Math. Soc. 80: 419–438. doi:10.1090/S0002-9904-1974-13434-8.
References
[edit]- Aletheia-Zomlefer, Soren Laing; Fukshansky, Lenny; Garcia, Stephan Ramon (2020). "The Bateman–Horn conjecture: Heuristic, history, and applications". Expositiones Mathematicae. 38 (4): 430–479. doi:10.1016/j.exmath.2019.04.005. ISSN 0723-0869.
- Tóth, László (January 2019). "On the Asymptotic Density of Prime k-tuples and a Conjecture of Hardy and Littlewood". Computational Methods in Science and Technology. 25: 143–138. arXiv:1910.02636. doi:10.12921/cmst.2019.0000033.

















